1 Introduction ................................................. 6
1.1 Background .............................................. 6
1.2 Some conventions ........................................ 6
1.3 The colored sl(N) link homology ......................... 6
1.4 Deformations and applications ........................... 8
1.5 Other approaches to the colored sl(N) link homology ..... 8
1.6 Outline of the proof .................................... 9
2 The MOY calculus ............................................ 11
2.1 The HOMFLYPT polynomial ................................ 11
2.2 MOY graphs ............................................. 11
2.3 The MOY graph polynomial ............................... 12
2.4 The MOY calculus ....................................... 13
2.5 The colored Reshetikhin-Turaev sl(N) polynomial ........ 16
3 Graded matrix factorizations ................................ 17
3.1 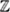 -pregraded and -pregraded and 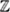 -graded linear spaces ................. 17
3.2 Graded modules over a graded -graded linear spaces ................. 17
3.2 Graded modules over a graded 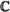 -algebra ................. 18
3.3 Graded matrix factorizations ........................... 19
3.4 Morphisms of graded matrix factorizations .............. 21
3.5 Elementary operations on Koszul matrix factorizations .. 25
3.6 Categories of homotopically finite graded matrix
factorizations ......................................... 32
3.7 Categories of chain complexes .......................... 34
4 Graded matrix factorizations over a polynomial ring ......... 36
4.1 Homogeneous basis ...................................... 36
4.2 Homology of graded matrix factorizations over R ........ 37
4.3 The Krull-Schmidt property ............................. 41
4.4 Yonezawa's lemma ....................................... 43
5 Symmetric polynomials ....................................... 46
5.1 Notations and basic examples ........................... 46
5.2 Partitions and linear bases for the space of
symmetric polynomials .................................. 48
5.3 Partially symmetric polynomials ........................ 50
5.4 The cohomology ring of a complex Grassmannian .......... 51
6 Matrix factorizations associated to MOY graphs .............. 52
6.1 Markings of MOY graphs ................................. 52
6.2 The matrix factorization associated to a MOY graph ..... 52
6.3 Direct sum decomposition (II) .......................... 57
6.4 Direct sum decomposition (I) ........................... 58
7 Circles ..................................................... 61
7.1 Homotopy type .......................................... 61
7.2 Module structure of the homology ....................... 62
7.3 Cycles representing the generating class ............... 66
8 Morphisms induced by local changes of MOY graphs ............ 70
8.1 A strategy in defining and comparing morphisms ......... 70
8.2 Bouquet move ........................................... 72
8.3 Circle creation and annihilation ....................... 73
8.4 Edge splitting and merging ............................. 74
8.5 Adjoint Koszul matrix factorizations ................... 76
8.6 General x-morphisms .................................... 82
8.7 Adding and removing a loop ............................. 93
8.8 Saddle move ............................................ 95
8.9 The first composition formula .......................... 96
8.10 The second composition formula ........................ 100
9 Direct sum decomposition (III) ............................. 111
9.1 Relating Г and Г0 ..................................... 111
9.2 Relating Г and Г1 ..................................... 117
9.3 Proof of Theorem 9.1 .................................. 120
10 Direct sum decomposition (IV) .............................. 126
10.1 Relating Г and Го ..................................... 127
10.2 Relating Г and Ti ..................................... 130
10.3 Homotopic nilpotency -algebra ................. 18
3.3 Graded matrix factorizations ........................... 19
3.4 Morphisms of graded matrix factorizations .............. 21
3.5 Elementary operations on Koszul matrix factorizations .. 25
3.6 Categories of homotopically finite graded matrix
factorizations ......................................... 32
3.7 Categories of chain complexes .......................... 34
4 Graded matrix factorizations over a polynomial ring ......... 36
4.1 Homogeneous basis ...................................... 36
4.2 Homology of graded matrix factorizations over R ........ 37
4.3 The Krull-Schmidt property ............................. 41
4.4 Yonezawa's lemma ....................................... 43
5 Symmetric polynomials ....................................... 46
5.1 Notations and basic examples ........................... 46
5.2 Partitions and linear bases for the space of
symmetric polynomials .................................. 48
5.3 Partially symmetric polynomials ........................ 50
5.4 The cohomology ring of a complex Grassmannian .......... 51
6 Matrix factorizations associated to MOY graphs .............. 52
6.1 Markings of MOY graphs ................................. 52
6.2 The matrix factorization associated to a MOY graph ..... 52
6.3 Direct sum decomposition (II) .......................... 57
6.4 Direct sum decomposition (I) ........................... 58
7 Circles ..................................................... 61
7.1 Homotopy type .......................................... 61
7.2 Module structure of the homology ....................... 62
7.3 Cycles representing the generating class ............... 66
8 Morphisms induced by local changes of MOY graphs ............ 70
8.1 A strategy in defining and comparing morphisms ......... 70
8.2 Bouquet move ........................................... 72
8.3 Circle creation and annihilation ....................... 73
8.4 Edge splitting and merging ............................. 74
8.5 Adjoint Koszul matrix factorizations ................... 76
8.6 General x-morphisms .................................... 82
8.7 Adding and removing a loop ............................. 93
8.8 Saddle move ............................................ 95
8.9 The first composition formula .......................... 96
8.10 The second composition formula ........................ 100
9 Direct sum decomposition (III) ............................. 111
9.1 Relating Г and Г0 ..................................... 111
9.2 Relating Г and Г1 ..................................... 117
9.3 Proof of Theorem 9.1 .................................. 120
10 Direct sum decomposition (IV) .............................. 126
10.1 Relating Г and Го ..................................... 127
10.2 Relating Г and Ti ..................................... 130
10.3 Homotopic nilpotency 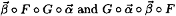 ........... 133
10.4 Graded dimensions of С(Г), С(Г0) and C(Г1) ............ 136
10.5 Proof of Theorem 10.1 ................................. 142
11 Direct sum decomposition (V) ............................... 144
11.1 The proof ............................................. 144
12 Chain complexes associated to knotted MOY graphs ........... 149
12.1 Change of base ring ................................... 151
12.2 Computing HomHMF(C(Гk2),*) ............................. 153
12.3 The chain complex associated to a colored crossing .... 157
12.4 A null homotopic chain complex ........................ 161
12.5 Explicit forms of the differential maps ............... 164
12.6 The graded Euler characteristic and the ........... 133
10.4 Graded dimensions of С(Г), С(Г0) and C(Г1) ............ 136
10.5 Proof of Theorem 10.1 ................................. 142
11 Direct sum decomposition (V) ............................... 144
11.1 The proof ............................................. 144
12 Chain complexes associated to knotted MOY graphs ........... 149
12.1 Change of base ring ................................... 151
12.2 Computing HomHMF(C(Гk2),*) ............................. 153
12.3 The chain complex associated to a colored crossing .... 157
12.4 A null homotopic chain complex ........................ 161
12.5 Explicit forms of the differential maps ............... 164
12.6 The graded Euler characteristic and the  2-grading .... 170
13 1nvariance under fork sliding .............................. 172
13.1 Notations used in the proof ........................... 174
13.2 Commutativity lemmas .................................. 176
13.3 Another look at decomposition (IV) .................... 180
13.4 Relating the differential maps C± and Ĉ(D1,1±) ......... 185
13.5 Relating the differential maps of Ĉ(D1,0±) and
Ĉ(D1,1±) .................................................... 187
13.6 Decomposing С(Гm,1) = С(Г'm) ........................... 191
13.7 Proof of Proposition 13.2 ............................. 196
14 Invariance under Reidemeister moves ........................ 206
14.1 Invariance under Reidemeister moves IIα, IIb and III ... 207
14.2 Invariance under Reidemeister move I .................. 208
References .................................................... 213
Two index charts .............................................. 216 2-grading .... 170
13 1nvariance under fork sliding .............................. 172
13.1 Notations used in the proof ........................... 174
13.2 Commutativity lemmas .................................. 176
13.3 Another look at decomposition (IV) .................... 180
13.4 Relating the differential maps C± and Ĉ(D1,1±) ......... 185
13.5 Relating the differential maps of Ĉ(D1,0±) and
Ĉ(D1,1±) .................................................... 187
13.6 Decomposing С(Гm,1) = С(Г'm) ........................... 191
13.7 Proof of Proposition 13.2 ............................. 196
14 Invariance under Reidemeister moves ........................ 206
14.1 Invariance under Reidemeister moves IIα, IIb and III ... 207
14.2 Invariance under Reidemeister move I .................. 208
References .................................................... 213
Two index charts .............................................. 216
|