 | Ceccherini-Silberstein T. Representation theory of the symmetric groups: the Okounkov-Vershik approach, character formulas, and partition algebras / T.Ceccherini-Silberstein, F.Scarabotti, F.Tolli. - Cambridge; New York: Cambridge University Press, 2010. - xv, 412 p.: ill. - (Cambridge studies in advanced mathematics; 121). - Ref.: p.402-408. - Ind.: p.409-412. - ISBN 978-0-521-11817-0
|
Preface page ................................................. xiii
1 Representation theory of finite groups ....................... 1
1.1 Basic facts ............................................. 1
1.1.1 Representations .................................. 1
1.1.2 Examples ......................................... 2
1.1.3 Intertwining operators ........................... 4
1.1.4 Direct sums and complete reducibility ............ 5
1.1.5 The adjoint representation ....................... 6
1.1.6 Matrix coefficients .............................. 7
1.1.7 Tensor products .................................. 8
1.1.8 Cyclic and invariant vectors .................... 10
1.2 Schur's lemma and the commutant ........................ 11
1.2.1 Schur's lemma ................................... 11
1.2.2 Multiplicities and isotypic components .......... 12
1.2.3 Finite dimensional algebras ..................... 14
1.2.4 The structure of the commutant .................. 16
1.2.5 Another description of HomG(W, V) ............... 18
1.3 Characters and the projection formula .................. 19
1.3.1 The trace ....................................... 19
1.3.2 Central functions and characters ................ 20
1.3.3 Central projection formulas ..................... 22
1.4 Permutation representations ............................ 27
1.4.1 Wielandt's lemma ................................ 27
1.4.2 Symmetric actions and Gelfand's lemma ........... 30
1.4.3 Frobenius reciprocity for a permutation
representation .................................. 30
1.4.4 The structure of the commutant of a permutation
representation .................................. 35
1.5 The group algebra and the Fourier transform ............ 37
1.5.1 L(G) and the convolution ........................ 37
1.5.2 The Fourier transform ........................... 42
1.5.3 Algebras of bi-K'-invariant functions ........... 46
1.6 Induced representations ................................ 51
1.6.1 Definitions and examples ........................ 51
1.6.2 First properties of induced representations ..... 53
1.6.3 Frobenius reciprocity ........................... 55
1.6.4 Mackey's lemma and the intertwining number
theorem ......................................... 57
2 The theory of Gelfand-Tsetlin bases ......................... 59
2.1 Algebras of conjugacy invariant functions .............. 59
2.1.1 Conjugacy invariant functions ................... 59
2.1.2 Multiplicity-free subgroups ..................... 64
2.1.3 Greenhalgebrus .................................. 65
2.2 Gelfand-Tsetlin bases .................................. 69
2.2.1 Branching graphs and Gelfand-Tsetlin bases ...... 69
2.2.2 Gelfand-Tsetlin algebras ........................ 71
2.2.3 Gelfand-Tsetlin bases for permutation
representations ................................. 75
3 The Okounkov-Vershik approach ............................... 79
3.1 The Young poset ........................................ 79
3.1.1 Partitions and conjugacy classes in 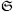 n .......... 79
3.1.2 Young frames .................................... 81
3.1.3 Young tableaux .................................. 81
3.1.4 Coxeter generators .............................. 83
3.1.5 The content of a tableau ........................ 85
3.1.6 The Young poset ................................. 89
3.2 The Young-Jucys-Murphy elements and a Gelfand-Tsetlin
basis for 6 ............................................ 91
3.2.1 The Young-Jucys-Murphy elements ................. 92
3.2.2 Marked permutations ............................. 92
3.2.3 Olshanskii's theorem ............................ 95
3.2.4 A characterization of the YJM elements .......... 98
3.3 The spectrum of the Young-Jucys-Murphy elements and
the branching graph of n .......... 79
3.1.2 Young frames .................................... 81
3.1.3 Young tableaux .................................. 81
3.1.4 Coxeter generators .............................. 83
3.1.5 The content of a tableau ........................ 85
3.1.6 The Young poset ................................. 89
3.2 The Young-Jucys-Murphy elements and a Gelfand-Tsetlin
basis for 6 ............................................ 91
3.2.1 The Young-Jucys-Murphy elements ................. 92
3.2.2 Marked permutations ............................. 92
3.2.3 Olshanskii's theorem ............................ 95
3.2.4 A characterization of the YJM elements .......... 98
3.3 The spectrum of the Young-Jucys-Murphy elements and
the branching graph of 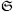 n ............................. 100
3.3.1 The weight of a Young basis vector ............. 100
3.3.2 The spectrum of the YJM elements ............... 102
3.3.3 Spec(n) = Cont(n) .............................. 104
3.4 The irreducible representations of n ............................. 100
3.3.1 The weight of a Young basis vector ............. 100
3.3.2 The spectrum of the YJM elements ............... 102
3.3.3 Spec(n) = Cont(n) .............................. 104
3.4 The irreducible representations of 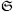 n ................. 110
3.4.1 Young's seminormal form ........................ 110
3.4.2 Young's orthogonal form ........................ 112
3.4.3 The Murnaghan-Nakayama rule for a cycle ........ 116
3.4.4 The Young seminormal units ..................... 118
3.5 Skew representations and the Murnhagan-Nakayama
rule .................................................. 121
3.5.1 Skew shapes .................................... 121
3.5.2 Skew representations of the symmetric group .... 123
3.5.3 Basic properties of the skew representations
and Pieri's rule ............................... 126
3.5.4 Skew hooks ..................................... 130
3.5.5 The Murnaghan-Nakayama rule .................... 132
3.6 The Frobenius-Young correspondence .................... 135
3.6.1 The dominance and the lexicographic orders
for partitions ................................. 135
3.6.2 The Young modules .............................. 138
3.6.3 The Frobenius-Young correspondence ............. 140
3.6.4 Radon transforms between Young's modules ....... 144
3.7 The Young rule ........................................ 145
3.7.1 Semistandard Young tableaux .................... 145
3.7.2 The reduced Young poset ........................ 148
3.7.3 The Young rule ................................. 150
3.7.4 A Greenhalgebra with the symmetric group ....... 153
4 Symmetric functions ........................................ 156
4.1 Symmetric polynomials ................................. 156
4.1.1 More notation and results on partitions ........ 156
4.1.2 Monomial symmetric polynomials ................. 157
4.1.3 Elementary, complete and power sums symmetric
polynomials .................................... 159
4.1.4 The fundamental theorem on symmetric
polynomials .................................... 165
4.1.5 An involutive map .............................. 167
4.1.6 Antisymmetric polynomials ...................... 168
4.1.7 The algebra of symmetric functions ............. 170
4.2 The Frobenius character formula ....................... 171
4.2.1 On the characters of the Young modules ......... 171
4.2.2 Cauchy's formula ............................... 173
4.2.3 Frobenius character formula .................... 174
4.2.4 Applications of Frobenius character formula .... 179
4.3 Schur polynomials ..................................... 185
4.3.1 Definition of Schur polynomials ................ 185
4.3.2 A scalar product ............................... 188
4.3.3 The characteristic map ......................... 189
4.3.4 Determinantal identities ....................... 193
4.4 The Theorem of Jucys and Murphy ....................... 199
4.4.1 Minimal decompositions of permutations as
products of transpositions ..................... 199
4.4.2 The Theorem of Jucys and Murphy ................ 204
4.4.3 Bernoulli and Stirling numbers ................. 208
4.4.4 Garsia's expression for χλ ..................... 213
5 Content evaluation and character theory of the symmetric
group ...................................................... 221
5.1 Binomial coefficients ................................. 221
5.1.1 Ordinary binomial coefficients: basic
identities ..................................... 221
5.1.2 Binomial coefficients: some technical
results ........................................ 224
5.1.3 Lassalle's coefficients ........................ 228
5.1.4 Binomial coefficients associated with
partitions ..................................... 233
5.1.5 Lassalle's symmetric function .................. 235
5.2 Taylor series for the Frobenius quotient .............. 238
5.2.1 The Frobenius function ......................... 238
5.2.2 Lagrange interpolation formula ................. 242
5.2.3 The Taylor series at infinity for the
Frobenius quotient ............................. 245
5.2.4 Some explicit formulas for the coefficients
сrλ(m) .......................................... 250
5.3 Lassalle's explicit formulas for the characters of
the symmetric group ................................... 252
5.3.1 Conjugacy classes with one nontrivial cycle .... 252
5.3.2 Conjugacy classes with two nontrivial cycles ... 254
5.3.3 The explicit formula for an arbitrary
conjugacy class ................................ 258
5.4 Central characters and class symmetric functions ...... 263
5.4.1 Central characters ............................. 264
5.4.2 Class symmetric functions ...................... 267
5.4.3 Kerov-Vershik asymptotics ...................... 271
6 Radon transforms, Specht modules and the Littlewood-
Richardson rule ............................................ 273
6.1 The combinatorics of pairs of partitions and the
Littlewood-Richardson rule ............................ 274
6.1.1 Words and lattice permutations ................. 274
6.1.2 Pairs of partitions ............................ 277
6.1.3 James' combinatorial theorem ................... 281
6.1.4 Littlewood-Richardson tableaux ................. 284
6.1.5 The Littlewood-Richardson rule ................. 290
6.2 Randon transforms, Specht modules and orthogonal
decompositions of Young modules ....................... 293
6.2.1 Generalized Specht modules ..................... 293
6.2.2 A family of Radon transforms ................... 298
6.2.3 Decomposition theorems ......................... 303
6.2.4 The Gelfand-Tsetlin bases for Mα revisited ..... 307
7 Finite dimensional *-algebras .............................. 314
7.1 Finite dimensional algebras of operators .............. 314
7.1.1 Finite dimensional *-algebras .................. 314
7.1.2 Burnside's theorem ............................. 316
7.2 Schur's lemma and the commutant ....................... 318
7.2.1 Schur's lemma for a linear algebra ............. 318
7.2.2 The commutant of a *-algebra ................... 320
7.3 The double commutant theorem and the structure of
a finite dimensional *-algebra ........................ 323
7.3.1 Tensor product of algebras ..................... 323
7.3.2 The double commutant theorem ................... 325
7.3.3 Structure of finite dimensional *-algebras ..... 327
7.3.4 Matrix units and central elements .............. 331
7.4 Ideals and representation theory of a finite
dimensional *-algebra ................................. 332
7.4.1 Representation theory of End(V) ................ 332
7.4.2 Representation theory of finite dimensional
*-algebras ..................................... 334
7.4.3 The Fourier transform .......................... 336
7.4.4 Complete reducibility of finite dimensional
*-algebras ..................................... 336
7.4.5 The regular representation of a *-algebra ...... 338
7.4.6 Representation theory of finite groups
revisited ...................................... 339
7.5 Subalgebras and reciprocity laws ...................... 341
7.5.1 Subalgebras and Bratteli diagrams .............. 341
7.5.2 The centralizer of a subalgebra ................ 343
7.5.3 A reciprocity law for restriction .............. 345
7.5.4 A reciprocity law for induction ................ 347
7.5.5 Iterated tensor product of permutation
representations ................................ 351
8 Schur-Weyl dualities and the partition algebra ............. 357
8.1 Symmetric and antisymmetric tensors ................... 357
8.1.1 Iterated tensor product ........................ 358
8.1.2 The action of n ................. 110
3.4.1 Young's seminormal form ........................ 110
3.4.2 Young's orthogonal form ........................ 112
3.4.3 The Murnaghan-Nakayama rule for a cycle ........ 116
3.4.4 The Young seminormal units ..................... 118
3.5 Skew representations and the Murnhagan-Nakayama
rule .................................................. 121
3.5.1 Skew shapes .................................... 121
3.5.2 Skew representations of the symmetric group .... 123
3.5.3 Basic properties of the skew representations
and Pieri's rule ............................... 126
3.5.4 Skew hooks ..................................... 130
3.5.5 The Murnaghan-Nakayama rule .................... 132
3.6 The Frobenius-Young correspondence .................... 135
3.6.1 The dominance and the lexicographic orders
for partitions ................................. 135
3.6.2 The Young modules .............................. 138
3.6.3 The Frobenius-Young correspondence ............. 140
3.6.4 Radon transforms between Young's modules ....... 144
3.7 The Young rule ........................................ 145
3.7.1 Semistandard Young tableaux .................... 145
3.7.2 The reduced Young poset ........................ 148
3.7.3 The Young rule ................................. 150
3.7.4 A Greenhalgebra with the symmetric group ....... 153
4 Symmetric functions ........................................ 156
4.1 Symmetric polynomials ................................. 156
4.1.1 More notation and results on partitions ........ 156
4.1.2 Monomial symmetric polynomials ................. 157
4.1.3 Elementary, complete and power sums symmetric
polynomials .................................... 159
4.1.4 The fundamental theorem on symmetric
polynomials .................................... 165
4.1.5 An involutive map .............................. 167
4.1.6 Antisymmetric polynomials ...................... 168
4.1.7 The algebra of symmetric functions ............. 170
4.2 The Frobenius character formula ....................... 171
4.2.1 On the characters of the Young modules ......... 171
4.2.2 Cauchy's formula ............................... 173
4.2.3 Frobenius character formula .................... 174
4.2.4 Applications of Frobenius character formula .... 179
4.3 Schur polynomials ..................................... 185
4.3.1 Definition of Schur polynomials ................ 185
4.3.2 A scalar product ............................... 188
4.3.3 The characteristic map ......................... 189
4.3.4 Determinantal identities ....................... 193
4.4 The Theorem of Jucys and Murphy ....................... 199
4.4.1 Minimal decompositions of permutations as
products of transpositions ..................... 199
4.4.2 The Theorem of Jucys and Murphy ................ 204
4.4.3 Bernoulli and Stirling numbers ................. 208
4.4.4 Garsia's expression for χλ ..................... 213
5 Content evaluation and character theory of the symmetric
group ...................................................... 221
5.1 Binomial coefficients ................................. 221
5.1.1 Ordinary binomial coefficients: basic
identities ..................................... 221
5.1.2 Binomial coefficients: some technical
results ........................................ 224
5.1.3 Lassalle's coefficients ........................ 228
5.1.4 Binomial coefficients associated with
partitions ..................................... 233
5.1.5 Lassalle's symmetric function .................. 235
5.2 Taylor series for the Frobenius quotient .............. 238
5.2.1 The Frobenius function ......................... 238
5.2.2 Lagrange interpolation formula ................. 242
5.2.3 The Taylor series at infinity for the
Frobenius quotient ............................. 245
5.2.4 Some explicit formulas for the coefficients
сrλ(m) .......................................... 250
5.3 Lassalle's explicit formulas for the characters of
the symmetric group ................................... 252
5.3.1 Conjugacy classes with one nontrivial cycle .... 252
5.3.2 Conjugacy classes with two nontrivial cycles ... 254
5.3.3 The explicit formula for an arbitrary
conjugacy class ................................ 258
5.4 Central characters and class symmetric functions ...... 263
5.4.1 Central characters ............................. 264
5.4.2 Class symmetric functions ...................... 267
5.4.3 Kerov-Vershik asymptotics ...................... 271
6 Radon transforms, Specht modules and the Littlewood-
Richardson rule ............................................ 273
6.1 The combinatorics of pairs of partitions and the
Littlewood-Richardson rule ............................ 274
6.1.1 Words and lattice permutations ................. 274
6.1.2 Pairs of partitions ............................ 277
6.1.3 James' combinatorial theorem ................... 281
6.1.4 Littlewood-Richardson tableaux ................. 284
6.1.5 The Littlewood-Richardson rule ................. 290
6.2 Randon transforms, Specht modules and orthogonal
decompositions of Young modules ....................... 293
6.2.1 Generalized Specht modules ..................... 293
6.2.2 A family of Radon transforms ................... 298
6.2.3 Decomposition theorems ......................... 303
6.2.4 The Gelfand-Tsetlin bases for Mα revisited ..... 307
7 Finite dimensional *-algebras .............................. 314
7.1 Finite dimensional algebras of operators .............. 314
7.1.1 Finite dimensional *-algebras .................. 314
7.1.2 Burnside's theorem ............................. 316
7.2 Schur's lemma and the commutant ....................... 318
7.2.1 Schur's lemma for a linear algebra ............. 318
7.2.2 The commutant of a *-algebra ................... 320
7.3 The double commutant theorem and the structure of
a finite dimensional *-algebra ........................ 323
7.3.1 Tensor product of algebras ..................... 323
7.3.2 The double commutant theorem ................... 325
7.3.3 Structure of finite dimensional *-algebras ..... 327
7.3.4 Matrix units and central elements .............. 331
7.4 Ideals and representation theory of a finite
dimensional *-algebra ................................. 332
7.4.1 Representation theory of End(V) ................ 332
7.4.2 Representation theory of finite dimensional
*-algebras ..................................... 334
7.4.3 The Fourier transform .......................... 336
7.4.4 Complete reducibility of finite dimensional
*-algebras ..................................... 336
7.4.5 The regular representation of a *-algebra ...... 338
7.4.6 Representation theory of finite groups
revisited ...................................... 339
7.5 Subalgebras and reciprocity laws ...................... 341
7.5.1 Subalgebras and Bratteli diagrams .............. 341
7.5.2 The centralizer of a subalgebra ................ 343
7.5.3 A reciprocity law for restriction .............. 345
7.5.4 A reciprocity law for induction ................ 347
7.5.5 Iterated tensor product of permutation
representations ................................ 351
8 Schur-Weyl dualities and the partition algebra ............. 357
8.1 Symmetric and antisymmetric tensors ................... 357
8.1.1 Iterated tensor product ........................ 358
8.1.2 The action of 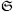 k on V k on V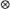 k ........................ 360
8.1.3 Symmetric tensors .............................. 361
8.1.4 Antisymmetric tensors .......................... 365
8.2 Classical Schur-Weyl duality .......................... 368
8.2.1 The general linear group GL(n, k ........................ 360
8.1.3 Symmetric tensors .............................. 361
8.1.4 Antisymmetric tensors .......................... 365
8.2 Classical Schur-Weyl duality .......................... 368
8.2.1 The general linear group GL(n, 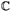 ) .............. 368
8.2.2 Duality between GL(n, ) .............. 368
8.2.2 Duality between GL(n, 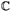 ) and ) and 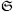 k ................ 374
8.2.3 Clebsch-Gordan decomposition and branching
formulas ....................................... 378
8.3 The partition algebra ................................. 384
8.3.1 The partition monoid ........................... 385
8.3.2 The partition algebra .......................... 391
8.3.3 Schur-Weyl duality for the partition algebra ... 393
References .................................................... 402
Index ......................................................... 409 k ................ 374
8.2.3 Clebsch-Gordan decomposition and branching
formulas ....................................... 378
8.3 The partition algebra ................................. 384
8.3.1 The partition monoid ........................... 385
8.3.2 The partition algebra .......................... 391
8.3.3 Schur-Weyl duality for the partition algebra ... 393
References .................................................... 402
Index ......................................................... 409
|
|